


Exposure Time Examples
Here are a few simple examples to illustrate how an integration time may be computed for point sources and diffuse sources. The flux values given here are for illustrative purposes only; you need to check the flux values if you are planning your own observations of one of these targets.
Spectroscopy of Diffuse Source (M86)
We want to observe M86, an elliptical galaxy in Virgo, using the G750M grating at a central wavelength setting of  c=6768 Å, the CCD detector and the 52 x 0.2 arcsec slit. Our aim is to calculate the H
c=6768 Å, the CCD detector and the 52 x 0.2 arcsec slit. Our aim is to calculate the H count rate in the central region of M86 and the expected signal-to-noise ratio per resolution element in an exposure time of 1 hour. M86 has an inhomogeneous surface brightness distribution in H
count rate in the central region of M86 and the expected signal-to-noise ratio per resolution element in an exposure time of 1 hour. M86 has an inhomogeneous surface brightness distribution in H and the line is well resolved with this grating. Let us consider a region with an H
and the line is well resolved with this grating. Let us consider a region with an H surface brightness of I
surface brightness of I = 1.16 x 10-15 erg sec-1 cm-2 Å-1 arcsec-2 (note the unit - it is not the entire H
= 1.16 x 10-15 erg sec-1 cm-2 Å-1 arcsec-2 (note the unit - it is not the entire H flux but the flux per unit wavelength interval) and a continuum surface brightness I
flux but the flux per unit wavelength interval) and a continuum surface brightness I = 2.32x 10-16 erg sec-1 cm-2 Å-1 arcsec-2. To derive the H
= 2.32x 10-16 erg sec-1 cm-2 Å-1 arcsec-2. To derive the H and continuum count rates from the source we use the formula from Chapter 13
and continuum count rates from the source we use the formula from Chapter 13
-
 = 1.14 x 1013 counts sec-1 pix
= 1.14 x 1013 counts sec-1 pix -1 pixs-1 per incident erg sec-1 cm-2 Å-1arcsec-2.
-1 pixs-1 per incident erg sec-1 cm-2 Å-1arcsec-2.
- N
 pix = 4 and Nspix = 2 (1 resolution element).
pix = 4 and Nspix = 2 (1 resolution element).
Using the equation given in the second section (Spectroscopy, Diffuse Source), we get the count rate C = 0.106 counts sec-1 in H and C=0.021 counts sec-1 in the continuum. Since we are interested in the properties of the H
and C=0.021 counts sec-1 in the continuum. Since we are interested in the properties of the H line, the H
line, the H counts constitute the signal, while both the H
counts constitute the signal, while both the H counts and the continuum counts contribute to the noise.
counts and the continuum counts contribute to the noise.
The sky background is negligible in comparison to the source, but the dark current (2.5 x 10-3 count sec-1 pixel-1 x 8 pixels = 0.020 count sec-1) and the read noise squared (19 electrons x 8 pixels x 3 reads = 465 counts, for cr-split=3) are important here. Substituting the numbers into the equation for signal-to-noise, we get:
To increase our signal-to-noise or decrease our exposure time, we can consider using on-chip binning. Let us bin 2 pixels in the spatial direction so that Nbin = 2. To allow adequate sampling of our new binned pixels, we leave N pix = 4, but set Nspix = 4, so Npix = 16 and then C = 0.212 for H
pix = 4, but set Nspix = 4, so Npix = 16 and then C = 0.212 for H and C=0.254 for the sum of H
and C=0.254 for the sum of H and continuum. To compute the time to achieve a signal-to-noise of 12 using this configuration, we use the full expression for the exposure time given on page 88, generalized to treat the line counts (for signal) and total counts (for noise) separately, and determine that roughly 30 minutes are needed in this configuration:
and continuum. To compute the time to achieve a signal-to-noise of 12 using this configuration, we use the full expression for the exposure time given on page 88, generalized to treat the line counts (for signal) and total counts (for noise) separately, and determine that roughly 30 minutes are needed in this configuration:
Spectroscopy of Solar-Analog Star P041-C
We wish to study the shape of the continuum spectrum of the solar-analog star P041-C from the near IR to the near UV. We wish to obtain spectroscopy with the CCD detector covering the entire useful spectral range from 2000 Å to 10,300 Å with gratings G230LB, G430L, and G750L. Since we require accurate photometry, we use the wide 52X0.5 slit. The goal is to reach a signal-to-noise ratio of 25 in the near UV (at 2300 Å), 100 in the blue, and 280 in the red. P041-C has V = 12.0.
The fluxes of P041-C at the desired wavelengths obtained from a spectrum of the Sun scaled from V = -26.75 to V = 12.0, are available via the web at:
http://www.stsci.edu/ftp/cdbs/cdbs2/calspec
G230LB
We illustrate the calculation of the exposure time for the G230LB grating. P041-C is found to have a flux of 1.7 x 10-15 erg sec-1 cm-2 Å-1 at 2300 Å.
We get the following values for G230LB from Chapter 13
-
 = 1.7 x 1014 counts sec-1 pix
= 1.7 x 1014 counts sec-1 pix -1per incident erg sec-1 cm-2 Å-1.
-1per incident erg sec-1 cm-2 Å-1.
- TA = 0.86 for the aperture throughput, taken from Chapter 13
-
 f = ~0.8.
f = ~0.8.
- Nspix=3, since ~80% of the point-source light is encircled with 3 pixels.
- N
 pix= 2, since two pixels resolve the LSF.
pix= 2, since two pixels resolve the LSF.
Using the equation on page 82, we calculate a point-source count rate of C = 0.34 counts sec-1 over Npix = 6 pixels for GAIN=1.
The source count rates can be compared with the background and detector dark current rates. Both background and detector rates are negligibly small for this setting; therefore we can neglect their contributions. Since we are aiming for a signal-to-noise ratio of 25, we can estimate that we must obtain 625 counts minimum. The read noise squared (~192 over 6 pixels for 2 readouts) must therefore be taken into account. Finally, since we are observing with the CCD in the near-UV, we must correct for the effect of the multiple-electron process (see page 88). This will cause the exposure time to be scaled approximately by Q, where Q is ~1.5 at 2300 Å. Using the STIS Exposure Time Calculator, we estimate the required time for S/N=25 is ~3560 seconds. To check that we indeed get S/N=25, we use the formula on page 87 as follows.
G750L and G430L
Exposure times for the two remaining wavelength settings can be calculated directly as time = signal-to-noise2 / C since the read noise, detector background, and sky background are negligible. As above, 3 pixels are taken to contain 80% of the flux. The results are summarized in Table 6.5.
Table 6.5: Low Resolution Spectroscopy of Solar Analog Star
| Grating |
G230LB |
G430L |
G750L  c=7751 |
| Wavelength (Angstroms) |
2300 |
5000 |
7800 |
| Flux (ergs sec-1 cm-2 Å-1) |
1.7x10-15 |
5.9x10-14 |
3.5x10-14 |
| Point Source Sensitivity (counts sec -1 pix  -1 -1 per ergs sec -1 cm -2 Å -1) |
1.7x1014 |
3.1x1015 |
5.0x1015 |
| Aperture throughput (TA) |
86% |
90% |
89% |
N  pix pix |
2 |
2 |
2 |
| Nspix to encircle 80% of PSF |
3 |
3 |
3 |
| C (counts sec-1 from source over Npix=6) |
0.34 |
240 |
240 |
| Signal-to-noise ratio desired |
25 |
100 |
280 |
| Total exposure time |
3560 seconds |
41 seconds |
330 seconds |
Extended Source with Flux in cgs units (NGC 6543): Imaging and Spectroscopy
Let us consider NGC 6543, the Cat's Eye planetary nebula, where the aim is to use the CCD to image using the [O II] filter, and to do spectroscopy both in the visible and in the UV.
Imaging
The aim is to get a signal-to-noise ratio of 30 using the [O II] filter. We know that NGC 6543 is about 6 times fainter in [O II] than in H , and its total flux at [O II] 3727 Å is ~4.4 x 10-11 erg sec-1 cm-2 contained within 1 Å. Since the radius of the object is about 10 arcsec, the average [O II] surface brightness is about 1.4 x 10-13 erg sec-1 cm-2 arcsec-2 Å-1.
, and its total flux at [O II] 3727 Å is ~4.4 x 10-11 erg sec-1 cm-2 contained within 1 Å. Since the radius of the object is about 10 arcsec, the average [O II] surface brightness is about 1.4 x 10-13 erg sec-1 cm-2 arcsec-2 Å-1.
We take:
-
 = 6.7 x 1011 counts sec-1 pix-1 Å-1per incident erg sec-1 cm-2 Å-1 arcsec-2 as given in Chapter 14.
= 6.7 x 1011 counts sec-1 pix-1 Å-1per incident erg sec-1 cm-2 Å-1 arcsec-2 as given in Chapter 14.
- We take Npix = 4 x 4 = 16, since a resolution element has radius of two pixels (see Chapter 14).
To calculate the count rate we use the equation on page 85 for diffuse sources and determine a per-pixel count rate of 0.094 counts sec-1 pixel-1 or a count rate C = 1.5 counts sec-1 over 16 pixels. The background and the dark current can be neglected. To get a signal-to-noise of 30 we need ~103 counts, so the read noise can also be neglected and we can use the simplified expression to calculate exposure time (see page 88). We obtain 103 counts in ~667 seconds. To allow post-observation removal of cosmic rays we use cr-split=2. We note that in each ~330 second exposure we predict a mean of ~31 counts pixel-1, and thus we are safely within the limits of the CCD full well.
Diffuse-Source Spectroscopy in the Visible and UV Regions
In the visible, the aim is to get a signal-to-noise of about 100 at  = 4861 Å, with the
= 4861 Å, with the G430M grating at a central wavelength setting of  c = 4961 Å, the CCD detector, and the
c = 4961 Å, the CCD detector, and the 52X0.1 arcsec slit. In the UV, the aim is to get a signal-to-noise ratio of about 20 at the C IV ~1550 Å line with the G140M grating at a central wavelength setting of  c = 1550 and the FUV-MAMA detector. To increase our signal-to-noise ratio in the UV, we use the
c = 1550 and the FUV-MAMA detector. To increase our signal-to-noise ratio in the UV, we use the 52X0.2 arcsec slit for the G140M spectroscopic observations.
Visible Region
NGC 6543 has an average H surface brightness of S(
surface brightness of S(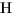
 ) ~ 8.37 x 10-13 erg sec-1 cm-2 Å-1 arcsec-2 at 4861 Å and has a radius of about 10 arcsec.
) ~ 8.37 x 10-13 erg sec-1 cm-2 Å-1 arcsec-2 at 4861 Å and has a radius of about 10 arcsec.
We take from Chapter 13.
-
 = 1.62 x 1012 counts sec-1 pix
= 1.62 x 1012 counts sec-1 pix -1 pixs-1 per incident erg sec-1 cm-2 Å-1 arcsec-2 for
-1 pixs-1 per incident erg sec-1 cm-2 Å-1 arcsec-2 for G430M.
- N
 pix = Nspix =2 since 2 pixels resolves the LSF and PSF.
pix = Nspix =2 since 2 pixels resolves the LSF and PSF.
Using the equation for diffuse sources on page 83, we derive a per-pixel count rate of 1.4 counts sec-1 pixel-1 and a count rate integrated over the four pixels of C = 5.4 counts sec-1 at 4861 Å from the astronomical source. The sky background and the detector background are much lower. To allow cosmic-ray removal in post-observation data processing, we use CR-SPLIT=3. To achieve a signal-to-noise of 100, we require a total of roughly 10,000 counts, so read noise should be negligible, even over 4 pixels and with nread = 3. We calculate the time required to achieve signal-to-noise of 100, using the simplified equation on page 88, and determine that we require roughly 30 minutes.
At a count rate of ~1 count sec-1 pixel-1 for 600 seconds per CR-SPLIT exposure, we are in no danger of hitting the CCD full-well limit.
UV Region
The C IV flux of NGC 6543 is ~ 2.5 x 10-12 erg sec-1 cm-2 arcsec-2 spread over ~1 Å. The line, with a FWHM ~ 0.4 Å, will be well resolved in the G140M configuration using the 52X0.2 slit.
We take from Chapter 13:
-
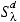 = 5.15 x109 counts sec-1 pix
= 5.15 x109 counts sec-1 pix -1 pixs-1 per incident erg sec-1 cm-2 Å-1 arcsec-2 for G140M at
-1 pixs-1 per incident erg sec-1 cm-2 Å-1 arcsec-2 for G140M at  =1550Å using the 0.2 arcsecond wide slit.
=1550Å using the 0.2 arcsecond wide slit.
- We take N
 pix = Nspix = 8, since the line emission is spread over the ~8 pixels of the slit width in dispersion, and we are willing to integrate flux along the slit to improve the signal-to-noise ratio.
pix = Nspix = 8, since the line emission is spread over the ~8 pixels of the slit width in dispersion, and we are willing to integrate flux along the slit to improve the signal-to-noise ratio.
Using the equation for diffuse sources on page 83, we determine a per-pixel peak count rate of ~0.013 counts sec-1 pixel-1 and a count-rate over the 64 pixels of C = 0.82 counts sec-1 at 1550 Å from the astronomical source. The sky and detector backgrounds are still negligible, and the read noise is zero for the MAMA detector so we can use the simplified equation for exposure time on page 88 directly. We determine that we require ~7 minutes.

We are well below the MAMA local linearity limit of 50 counts sec-1 pixel-1. Even assuming the nebula evenly illuminates the full 28 arcseconds of the long slit, we are well below the global absolute and linearity limits, since the flux from the nebula is concentrated in the C IV emission line. Then the global count rate, if the source fully fills the slit in the spatial direction, is given roughly by (0.015 x 8 x 1024) << 200,000 counts sec-1. Finally, we are well below the MAMA 16 bit buffer limit of a maximum of 65,536 counts pixel-1 integrated over the exposure duration.
Echelle Spectroscopy of a Bright Star with Large Extinction (Sk -69° 215)
The aim here is to do high-resolution echelle spectroscopy of an O5 star in the LMC (such as Sk -69° 215) at 2500 Å, using the E230H grating at a central wavelength of  c = 2513 Å and using the
c = 2513 Å and using the 0.2X0.09 arcsec slit. The aim is to get a signal-to-noise ratio of about 50 from photon statistics. We will assume that the exact UV flux of the star is unknown and we need to estimate it from the optical data. This calculation of the stellar flux at 2500 Å involves 2 steps:
- Calculation of the dereddened flux at 5500 Å.
- Calculation of predicted flux at 2500 Å taking reddening with standard extinction and stellar models into account.
Dereddened Magnitude and Prediction of 2500 Å Flux
We assume that it is an O5 V star with V = 11.6 (its exact spectral type is slightly uncertain). The expected B - V value from such a star is -0.35, whereas the observed B - V is -0.09; we thus get E(B - V) = 0.26 mag.
We assume all the extinction to be due to the LMC, and use the appropriate extinction law (Koornneef and Code, ApJ, 247, 860, 1981). The total visual extinction is then R x E(B - V) = 3.1 x 0.26 = 0.82, leading to an unreddened magnitude of V0 = 10.78. The corresponding flux at 5500 Å (using the standard zero point where V = 0 corresponds to F(5500 Å) = 3.55 x 10-9 erg sec-1 cm-2 Å-1) is F(5500 Å) = 1.73 x 10-13 erg sec-1 cm-2 Å-1.
The model atmosphere of Kurucz predicts F(2500 Å) / F(5500 Å) = 17.2 for an O5 star, which leads to a flux of F2500 Å = 2.98 x 10-12 erg sec-1 cm-2 Å-1 at 2500 Å for the unreddened star. Reddening will diminish this flux by a factor of 10-0.4xA(2500 Å), where the absorption at 2500 Å can be determined from the extinction curve; the result in this case is A (2500 Å) = 1.3. Thus the predicted flux of this star at 2500 A is 9.0 x 10-13 erg sec-1 cm-2 Å-1.
Exposure-Time Calculation
We take from Chapter 13:
-
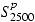 = 2.9 x 1011 counts sec-1 pix
= 2.9 x 1011 counts sec-1 pix -1per incident erg sec-1 cm-2 Å-1 for
-1per incident erg sec-1 cm-2 Å-1 for E230H.
- TA = 0.659 for the aperture throughput.
-
 f = 0.8 for the encircled energy.
f = 0.8 for the encircled energy.
- N
 pix= 2, since two pixels resolve the LSF.
pix= 2, since two pixels resolve the LSF.
- Nspix= 3, since 80% of the point source light is encircled by 3 pixels.
Using the equation for point sources on page 82, we determine a total count rate from the star of C = 0.3 counts sec-1 over 6 pixels. From Chapter 13 we see that ~22 percent of the point-source flux will be contained within the peak pixel. Thus the peak per pixel count rate will be approximately 0.3 x 0.22 / (0.8 x 2) = 0.045 counts sec-1 pixel-1 and well within the local linear counting regime. We can use the information that we register ~0.3 counts sec-1 for every two pixels in the dispersion direction to estimate the global count rate (over the entire detector) as follows. Each order contains ~1024 pixels, and the E230H grating at the central wavelength setting of 2513 Å covers 33 orders (see Chapter 13). A rough estimate of the global count rate is thus ~33 x 512 x 0.3/ 0.8 ~6400 count sec-1 and we are well within the linear range.
To calculate the integration time, we can ignore both the sky background and the detector dark current which are several orders of magnitude fainter than the source. To achieve a signal-to-noise ratio of 50 we then require ~2500 counts which would take a total of ~2.3 hours. Fortunately, this is a CVZ target!
Imaging a Faint Stellar Source
Consider a case where the aim is to image a faint (V = 28), A-type star with the clear filter and the CCD detector. We want to calculate the integration time required to achieve a signal-to-noise ratio of 5. The count rate from the source is 0.14 counts sec-1 distributed over about 25 pixels using the information in Chapter 14. If we assume the background to be "typical high" ( Table 6.4), the count rate due to the background integrated over the bandpass is ~ 0.22 counts sec-1 pixel-1 or 5.5 counts sec-1 in 25 pixels (and the detector dark rate is 64 times lower). We will need to be able to robustly distinguish cosmic rays if we are looking for faint sources, so we will use CR-SPLIT=4. We use the STIS Exposure Time Calculator to estimate the required exposure time to be 7313 seconds. To reproduce the numbers given by the ETC, we use the equation on page 87:

Alternately, we could have requested LOW-SKY (see Sky Background), since these observations are sky-background limited. In that case the sky background integrated over the bandpass produces ~0.018 counts sec-1 pixel-1 to which we add the detector dark current to get a total background of 0.020 counts sec-1 pixel-1. Using the full equation for exposure time again, we then determine that we require only ~30 minutes. This option is preferable to perform this experiment. To check the S/N, we use the equation on page 87:
Time-Tag Observations of a Flare Star (AU Mic)
Suppose the aim is to do TIME-TAG observations of a flare star such as AU Mic, in the hydrogen Lyman- 1216 Å line (see "MAMA TIME-TAG Mode" on page 219). We wish to observe it with the
1216 Å line (see "MAMA TIME-TAG Mode" on page 219). We wish to observe it with the G140M grating, the MAMA detector and a 0.2 arcsec slit. AU Mic has V = 8.75, the intensity of its the Ly- line is about 6 (± 3) x 10-12 erg sec-1 cm-2 Å-1, and the width (FWHM) of the line is about 0.7 (± 0.2) Å. We will assume that during bursts, the flux might vary by a factor of 10, so that the line flux may be up to 60 x 10-12 erg cm-2 sec-1 Å-1. AU Mic is an M star and its ultraviolet continuum is weak and can be neglected.
line is about 6 (± 3) x 10-12 erg sec-1 cm-2 Å-1, and the width (FWHM) of the line is about 0.7 (± 0.2) Å. We will assume that during bursts, the flux might vary by a factor of 10, so that the line flux may be up to 60 x 10-12 erg cm-2 sec-1 Å-1. AU Mic is an M star and its ultraviolet continuum is weak and can be neglected.
We use from Chapter 13:
-
 = 2.30x 1012 counts sec-1 pix
= 2.30x 1012 counts sec-1 pix -1per incident erg sec-1 cm-2 Å-1.
-1per incident erg sec-1 cm-2 Å-1.
- Aperture throughput TA = 0.6.
- Encircled energy
 f = 0.8.
f = 0.8.
- Nspix = 10.
- Derive N
 pix= 14 since the line FWHM is ~ 0.7 Å and the dispersive plate scale for G140M is 0.05 Å pixel-1.
pix= 14 since the line FWHM is ~ 0.7 Å and the dispersive plate scale for G140M is 0.05 Å pixel-1.
Plugging these values into the point-source equation on page 82, we get C = 927 counts sec-1 over 10 x 14 pixels, or ~1160 counts sec-1 from the source during a burst (taking  f = 1.0). This is well below the MAMA
f = 1.0). This is well below the MAMA TIME-TAG global linearity limit of 30,000 count sec-1 and the continuous observing limit of 26,000 count sec-1. The line is spread over 14 pixels in dispersion and roughly only 10% of the flux in the dispersion direction falls in the peak pixel; thus the peak per-pixel count rate, Pcr, is roughly 927 / (14 x 10) = 7 counts sec-1 pixel-1, and we are not near the MAMA local linearity limit.
For a TIME-TAG exposure, we need to determine our maximum allowed total observation time, which is given by 6.0 x 107/ C seconds or roughly 1079 minutes = 18 hours. For Phase II only, we will also need to compute the value of the buffer-time parameter, which is the time in seconds to reach 2 x 106 counts, in this case 2157 seconds (=2x106/927).


 c=6768 Å, the CCD detector and the 52 x 0.2 arcsec slit. Our aim is to calculate the H
c=6768 Å, the CCD detector and the 52 x 0.2 arcsec slit. Our aim is to calculate the H count rate in the central region of M86 and the expected signal-to-noise ratio per resolution element in an exposure time of 1 hour. M86 has an inhomogeneous surface brightness distribution in H
count rate in the central region of M86 and the expected signal-to-noise ratio per resolution element in an exposure time of 1 hour. M86 has an inhomogeneous surface brightness distribution in H and the line is well resolved with this grating. Let us consider a region with an H
and the line is well resolved with this grating. Let us consider a region with an H surface brightness of I
surface brightness of I = 1.16 x 10-15 erg sec-1 cm-2 Å-1 arcsec-2 (note the unit - it is not the entire H
= 1.16 x 10-15 erg sec-1 cm-2 Å-1 arcsec-2 (note the unit - it is not the entire H flux but the flux per unit wavelength interval) and a continuum surface brightness I
flux but the flux per unit wavelength interval) and a continuum surface brightness I = 2.32x 10-16 erg sec-1 cm-2 Å-1 arcsec-2. To derive the H
= 2.32x 10-16 erg sec-1 cm-2 Å-1 arcsec-2. To derive the H and continuum count rates from the source we use the formula from Chapter 13
and continuum count rates from the source we use the formula from Chapter 13



 = 1.14 x 1013 counts sec-1 pix
= 1.14 x 1013 counts sec-1 pix

 = 1.7 x 1014 counts sec-1 pix
= 1.7 x 1014 counts sec-1 pix f = ~0.8.
f = ~0.8.

 , and its total flux at [O II] 3727 Å is ~4.4 x 10-11 erg sec-1 cm-2 contained within 1 Å. Since the radius of the object is about 10 arcsec, the average [O II] surface brightness is about 1.4 x 10-13 erg sec-1 cm-2 arcsec-2 Å-1.
, and its total flux at [O II] 3727 Å is ~4.4 x 10-11 erg sec-1 cm-2 contained within 1 Å. Since the radius of the object is about 10 arcsec, the average [O II] surface brightness is about 1.4 x 10-13 erg sec-1 cm-2 arcsec-2 Å-1.
 = 6.7 x 1011 counts sec-1 pix-1 Å-1per incident erg sec-1 cm-2 Å-1 arcsec-2 as given in
= 6.7 x 1011 counts sec-1 pix-1 Å-1per incident erg sec-1 cm-2 Å-1 arcsec-2 as given in 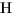
 = 1.62 x 1012 counts sec-1 pix
= 1.62 x 1012 counts sec-1 pix
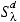 = 5.15 x109 counts sec-1 pix
= 5.15 x109 counts sec-1 pix
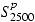 = 2.9 x 1011 counts sec-1 pix
= 2.9 x 1011 counts sec-1 pix

 = 2.30x 1012 counts sec-1 pix
= 2.30x 1012 counts sec-1 pix